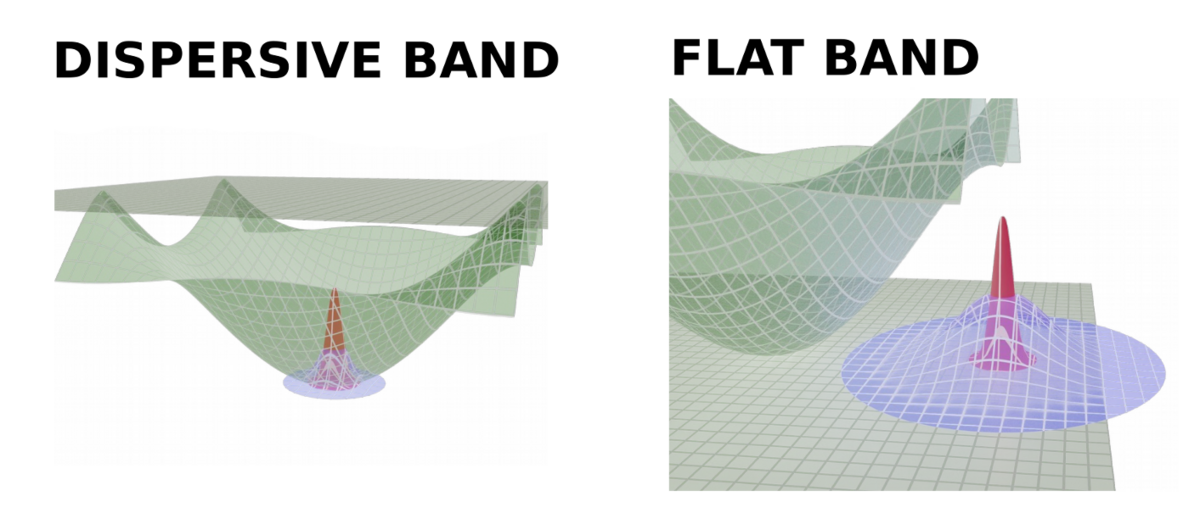
Quantum phenomena arising from interactions are exceptionally strong when particles do not have kinetic energy, which happens in flat energy bands. Aleksi Julku and Georg M. Bruun have together with Päivi Törma (Aalto University) in a work published in Physical Review Letters shown that a Bose-Einstein condensate (BEC), a state of matter where quantum particles behave as if they were a single macroscopic body, is possible in such flat band – if the quantum geometry of the band is right. Quantum geometry gives information concerning distances in the quantum world much like the usual geometry describing classical objects that one learns in school. In a BEC, atoms or light usually gather to the minimum of the energy landscape, which in a flat band is trying to contain water on a flat surface – indeed it would be impossible in the original scenario of Einstein and Bose. Previous work has shown that interactions add a small minimum to the flat band where the BEC can form. However, a repulsive interaction between the particles tend to kick them out of the condensate, and it was previously not known when it is stable against such quantum fluctuations. The new work shows that this crucial stability is guaranteed if the minimum point has a non-zero quantum distance to the other points in the flatland. This means that the promise of strong interaction effects in flat bands can indeed be utilized for creating new quantum phenomena and applications based on BEC.   

The article can be found here: https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.127.170404